Research Highlights

Galaxy clusters are the most massive collapsed objects in the Universe. They are exquisit astrophysical laboratories and very sensitive cosmological probes. We have performed multi-physics hydrodynamical simulations with the RAMSES code to study their properties and their evolution. In these simulations, we find that the cool-core/non-cool-core dichotomy of observed clusters is reproduced naturally in a cosmological environment. Cool cores are destroyed by low angular momentum major mergers. We also compare in detail the simulations with X-ray, SZ-, lensing results as well as constraints from galaxy properties. Another paper has just been accepted by MNRAS, where we studied the covariance between the stellar and the gaseous content of these massive systems. More to come soon!

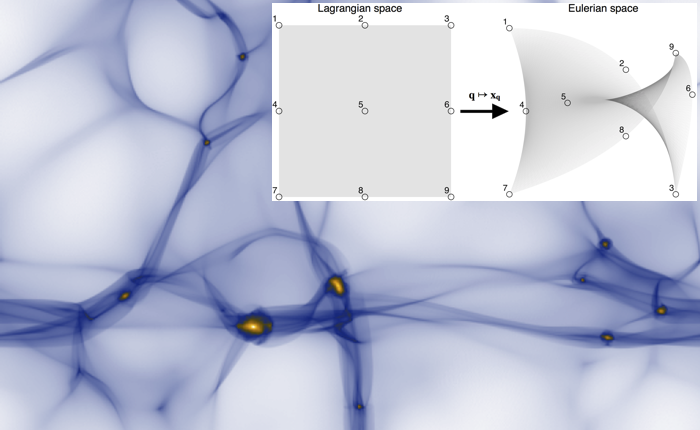
Dark matter numerical simulations and the N-body method are essential for understanding how structure forms and evolves in the Universe. However, the discrete nature of N-body simulations can affect its accuracy when modelling collisionless systems. We introduce a new approach to simulate the gravitational evolution of cold collisionless fluids by solving the Vlasov-Poisson equations in terms of adaptively refineable "Lagrangian phase space elements". These geometrical elements are piecewise smooth maps between three-dimensional Lagrangian space and six-dimensional Eulerian phase space and approximate the continuum structure of the distribution function. They allow for dynamical adaptive splitting to follow the evolution even in regions of very strong mixing. We discuss various test problems which demonstrate the correctness and performance of our method. We show that it has several advantages compared to standard N-body algorithms by i) explicitly tracking the fine-grained distribution function, ii) naturally representing caustics, iii) providing an arbitrarily regular density field defined everywhere in space, iv) giving a smooth and regular gravitational potential field, thus eliminating the need for any type of ad-hoc force softening. Finally, we illustrate the feasibility of using our method for cosmological studies by simulating structure formation in a warm dark matter cosmology. We show that spurious collisionality and discreteness noise of N-body methods are both strongly suppressed, which eliminates artificial fragmentation of filaments while providing access to the full deterministic evolution of the fluid in phase space. Therefore, we argue that our new approach improves on the N-body method when simulating self-gravitating cold and collisionless fluids, and is the first method that allows to explicitly follow the fine-grained evolution in six-dimensional phase space.

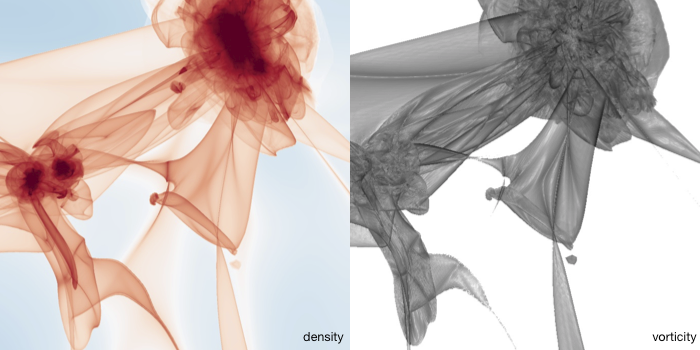
Understanding the velocity field is very important for modern cosmology: it gives insights to structure formation in general, and also its properties are crucial ingredients in modelling redshift-space distortions and in interpreting measurements of the kinetic Sunyaev-Zeldovich effect. Unfortunately, characterising the velocity field in cosmological N-body simulations is very much complicated by two facts: i) The velocity field becomes manifestly multi-valued after shell-crossing and has discontinuities at caustics. This is due to the collisionless nature of dark matter. ii) N-body simulations sample the velocity field only at a set of discrete locations, with poor resolution in low-density regions. In this paper, we discuss how the associated problems can be circumvented by using a phase-space interpolation technique. This method provides extremely accurate estimates of the cosmic velocity fields and its derivatives, which can be properly defined without the need of the arbitrary "coarse-graining" procedure commonly used. We explore in detail the configuration-space properties of the cosmic velocity field on very large scales and in the highly nonlinear regime. In particular, we characterise the divergence and curl of the velocity field, present their one-point statistics, analyse the Fourier-space properties and provide fitting formulae for the velocity divergence bias relative to the non-linear matter power spectrum. We furthermore contrast some of the interesting differences in the velocity fields of warm and cold dark matter models.